Why Chaos?

---

Conditions for Chaos

Necessary conditions for a set of evolution equations (assumed to be first order differential equations) to show chaos are:

• There must be at least three variables and equations
• There must be some nonlinearity

Kirchoff's equations for the circuit, involving equations for the voltages v₁ across C₁, v₂ across C₂, and the current i through the inductor L satisfy these requirements

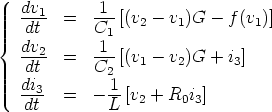
• The requirement of 3 equations is given by the inclusion of three reactive elements C₁, C₂, and L.
• The nonlinearity in the circuit comes from the piecewise linear effective negative resistance f(v_R), built using two op-amps and six resistors, as explained here. Thus the only nonlinearity in the effective circuit is the kink in the i-v characteristic f(v_R).

Oscillations

The equilibrium points are the intersections of the "load line" of slope -1/(R + R₀) with the Chua's diode characteristic f(v_R). Apart the equilibrium point at the origin, there are actually two possibilities, at positive and negative values. For suitable circuit values these stationary solutions are unstable and lead to growing oscillations about the equilibrium point. Since f(v_R) is locally linear, the amplitude of the oscillations will continue to grow, until the voltage somewhere in the cycle reaches the kink in f(v_R) at B_P. Then the oscillation growth can eventually saturate.

A typical observation will be oscillations where the voltage v₁ oscillates about one of the two equilibrium point V₀ (or -V₀ ) with an amplitude large enough to swing the voltage past the kink at B_P (or - B_P ).

Chaos

It is hard to predict the effect of the non-linearity: Does the periodic orbit persist or does it break down to chaos? Which of the routes to chaos occurs? What is the nature of the chaotic dynamics?

Observation on the physical circuit or the simulation shows that the periodic orbit undergoes several period doubling bifurcations, and then becomes noisy. At first the noise is weak (as expected for the period doubling route to chaos), perturbing the main oscillation about V₀ , but eventually, as parameters change, the dynamics begins to switch randomly back and forth between oscillations about V₀ and then -V₀ . In this regime the chaotic dynamics is quite reminiscent of Lorenz chaos. In fact, if we rescale the variables the equations

are quite reminiscent of the Lorenz equations, except that the product nonlinearities there are replaced by the piecewise linear function of a single variable here.

---

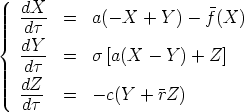
Last updated:

---

[Return to Home Page]